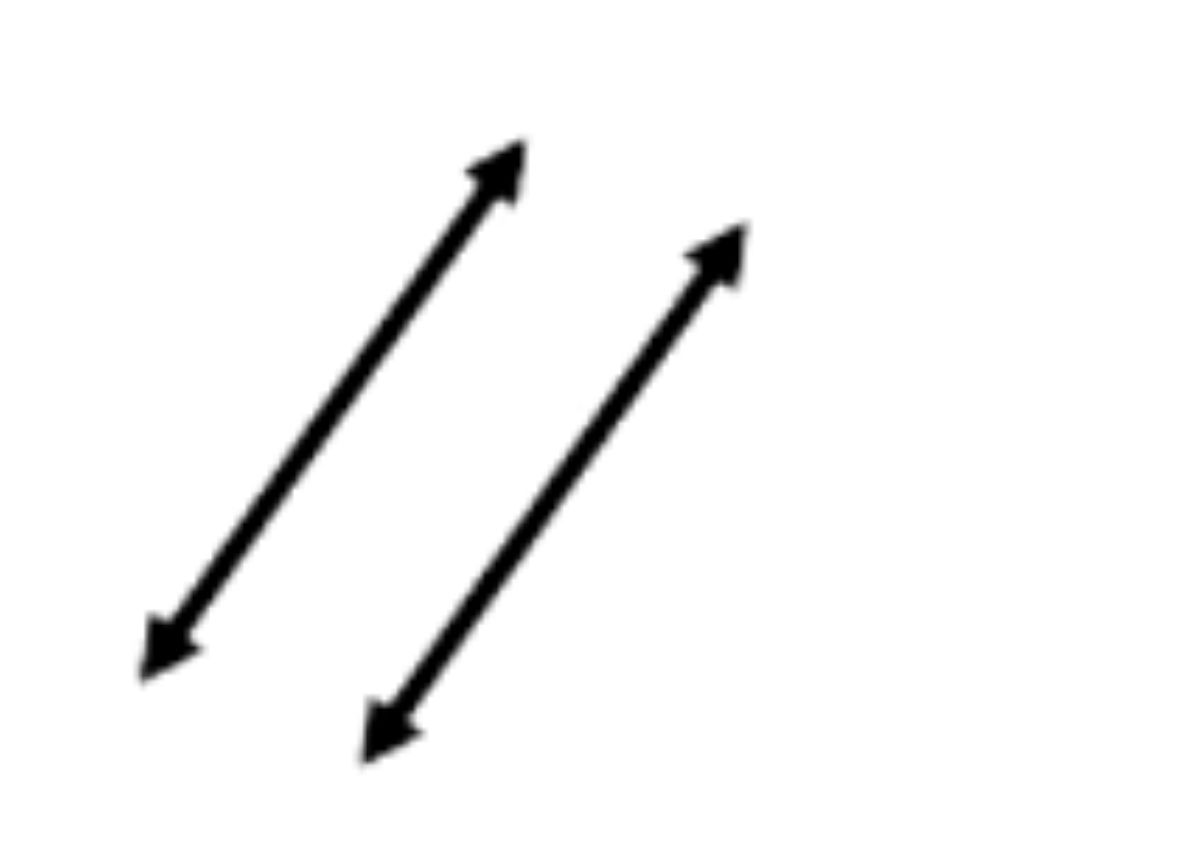
The lines that do not intersect or meet each other at any point in a plane are termed parallel lines. Parallel lines are non-intersecting lines and always stay apart from each other. It is also said that parallel lines meet at infinity.
Definition of Parallel Lines
Parallel lines in geometry can be defined as two straight lines in the plane that are at equal distance from each other and never intersects no matter how much they are extended. Parallel lines are represented by the symbol “||”.
Representation of Parallel Lines
Two parallel lines AB and CD are represented as AB||CD. This means line AB is parallel to line CD. In the given plane, infinite parallel lines can be drawn to AB and CD.
What are the Different Types of Angles Formed When Transversal Line Intersect Parallel Line?
When transversal line intersects two parallel lines, the following pair of angles are formed:
- Corresponding angles
- Alternate interior angles
- Alternate exterior angles
- Vertically opposite angles
- Linear Pair
Real-Life Applications of Parallel Lines
We observe parallel lines in the following things:
- Railway Track
- Cricket Stumps
- Racing Track
- Fork Lines
- Steps of a Ladder
- Rail Bars
- Keys of a Piano
- Pins of a Plug
- Markings on Road
- Zebra Crossing
- Edges of A Ruler
How To Identify Parallel Lines?
- Two lines intersected by a transversal line are termed parallel lines when corresponding angles are equal.
- Two lines intersected by a transversal line are termed parallel lines when alternate interior angles are equal.
- Two lines intersected by a transversal line are termed parallel lines when alternate exterior angles are equal.
- Two lines intersected by a transversal line are termed parallel lines when sum of consecutive interior angles is 180 degrees.
- Two lines intersected by a transversal line are termed parallel lines when sum of consecutive exterior angles is 180 degrees.
What is Geometry?
Geometry is regarded as the oldest part of Mathematics that is concerned with the property of space that is related to the shape, size, distance, and relative position of figures. A mathematician whose area of work is in Geometry is known as Geometer. The need for Geometry is not only limited to the study of a flat surface (known as plane geometry) and three-dimensional objects (known as solid Geometry).
What Are The Different Types of Geometry?
The different types of Geometry are:
- Eucledian Geometry: It is the most common type of Geometry that is generally taught at the primary level. Euclidean Geometry is described by Euclidean in detail in ‘Element’ which is one of the bases of Mathematics. The impact of ‘Element’ was so immense that no other kind of Geometry was almost used for the next 2000 years.
- Non-Euclidean Geometry: This type of Geometry is an extension to Euclid Geometry which is mostly observed in three-dimensional objects. The other name of Non-Euclidean Geometry is hyperbolic geometry, spherical geometry, elliptic geometry, and many more. This type of Geometry represents how most know theorem ‘Sum of the angles of a triangle is very different in 3-D space.’
- Analytic Geometry: In Analytic Geometry, we study geometric figures and construction using a coordinate system. The most commonly used coordinate systems are Cartesian, Polar, and Parametric systems.
- Differential Geometry: In differential Geometry, we study planes, lines, and surfaces in a three-dimensional space with the principles of integrals and differential calculus. This type of Geometry emphasizes several problems like contact surface, geodesics, complex manifolds, and several others. Geodesic is termed as the shortest distance between two points on the surface of a sphere. The use of Differential Geometry ranges from engineering problems to the computation of gravitational fields.
Read more interesting articles at Hub Blogging